Before starting

• Thank the organizers (applause).
• Joint work with E. Artal-Bartolo and S. Isaza.
• Please ask!
• Warning: topology inside. Might contain traces of singularities.

Motivation

Take an isolated surface singularity.

By Milnor fibration theorem, the germ is the topological cone over the link.

• Take a resolution of singularities, with exceptional divisor \(E\).
• The link becomes the boundary of a regular neighborhood of \(E\).
• It can be decomposed in pieces corresponding to the components of \(E\).
• Each piecse fibers over a component of \(E\), with fiber \(\mathbb{S}^1\)
• At each normal crossing, two pieces must be glued:
  • The fiber of one piece corresponds to a section of the second.

\(\mathbb{S}^1\)-bundles over (closed,oriented) surfaces.

Let \(\pi: M \rightarrow \Sigma\) be a \(\mathbb{S}^1\) bundle

• Take a disk \(D\) on the surface \(\Sigma\).
• Over \(D\), the bundle is a product, take a section \(s_1\).
• Over \(\Sigma\setminus D\), it is also a product, with section \(s_2\).
• \(s_1(\partial D)\) and \(s_2(\partial (\Sigma \setminus D))\) are two curves in a torus \(\mathbb{S}^1 \times \mathbb{S}^1\)
• The intersection number of these two curves is called the Euler number of the bundle

The Euler number of an \(\mathbb{S}^1\) bundle

• Is well defined
• Classifies the bundle
• It is aditive
• In the case of regular neighborhoods of curves in a surface, coincides with the self intersection

Plumbing

Given two \(\mathbb{S}^1\) bundles \(\pi_1: M_1 \rightarrow \Sigma_1\), \(\pi_2: M_2 \rightarrow \Sigma_2\)

• Consider a disk \(D_i\) in each \(\Sigma_i\) and the trivialization \(\pi_i^{-1}(D_i)=D_i\times \mathbb{S}^1\)
• In \(\partial D_i \times \mathbb{S}^1\) we have a fiber \(F_i\) and a section \(S_i\)
• Take \(\pi_i^{-1}(\Sigma_i\setminus D_i)\), they have a torus as boundary.
• Glue the tori, in such a way that \(F_1\cong S_2\), \(F_2\cong S_1\).
• We get a new manifold.
• This operation is called plumbing.

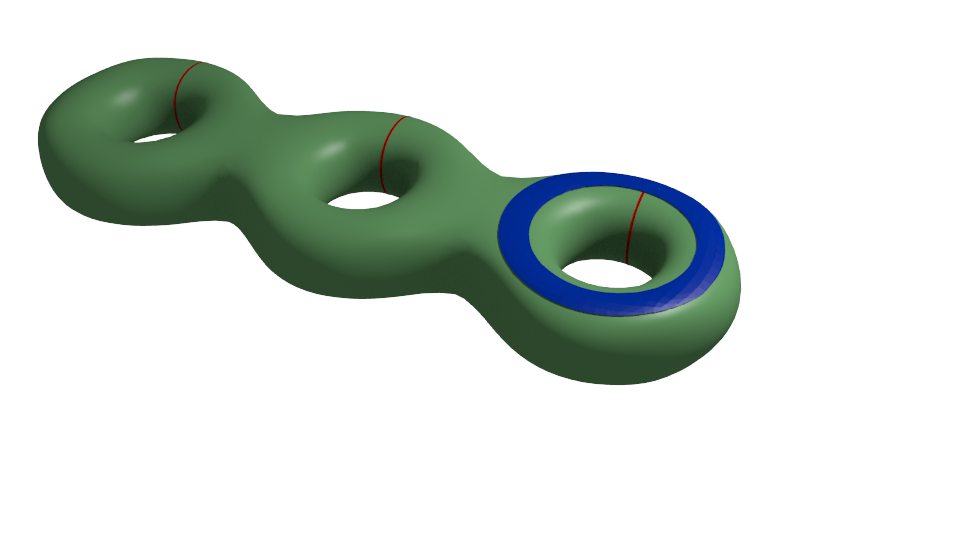
Graph manifolds

Are obtained by several plumbings

• They can be described by a decorated graph:
  • Each vertex is a bundle.
  • Each edge is a plumbing.
  • The links of a surface singularity are graph manifolds, described by the resolution graph.

Handle bodies

Are the result of adding handles to closed balls (equivalently, identifying pairs of disks in the boundary)

There are special curves that identify the handles (cutting curves).

Heegaard splittings

Two handle bodies of the same genus can be glued along the boundary, obtaining a closed \(3\)-manifold.

Every \(3\)-manifold can be obtained in this way (although not in a unique way).

The gluing surface, together with the two systems of cutting curves is called the Heegaard diagram of the splitting.

Heegaard-Floer homology is defined after such diagrams.

Previous lemmas

Let \(\mathbb{T}\) be a solid torus, \(c\) a closed curve in \(\partial \mathbb{T}\) isotopic to its core. \(M\) a \(3\)-manifold with boundary, and \(s\) a closed curve in \(\partial M\). Consider \(A_c\), \(A_s\) small neighborhoods in the boundaries of \(c\) and \(s\).

Lemma: The result of gluing \(\mathbb{T}\) with \(M\) through an orientation reversing homeomorphism \(A_c\to A_s\) is homeomorphic to \(M\).

This operation is called a float gluing

Proof of lemma

Heegaard diagrams of \(\mathbb{S}^1\) bundles

Lemma: Let \(\Sigma'\) be an oriented surface of genus \(g\) with one boundary component. Then \(\Sigma'\times I\) is a handle body of genus \(2g\).

Heegaard diagrams of \(\mathbb{S}^1\) bundles

Heegaard diagrams of \(\mathbb{S}^1\) bundles

Heegaard diagrams of \(\mathbb{S}^1\) bundles

Lemma: Let \(\Sigma'\) be an oriented surface of genus \(g\) with \(n\) boundary components. Then \(\Sigma'\times I\) is a handle body of genus \(2g+n-1\).

Heegaard diagrams of \(\mathbb{S}^1\) bundles

Lemma: Let \(\Sigma'\) be an oriented surface of genus \(g\) with \(n\) boundary components. Then \(\Sigma'\times I\) is a handle body of genus \(2g+n-1\).

Heegaard diagrams of \(\mathbb{S}^1\) bundles

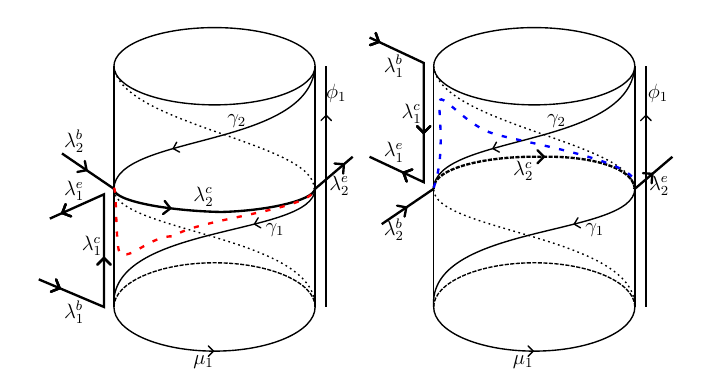
Heegaard splittings of \(\mathbb{S}^1\) bundles

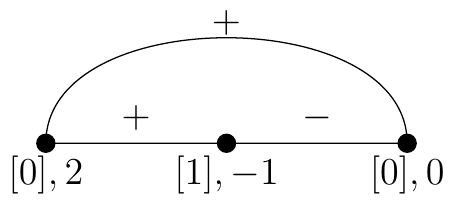
Let's start with a \((g,e)\) \(\mathbb{S}^1\) bundle \(\pi\).

• Take one or more disjoint disks \(D_1,\ldots, D_n\) in \(\Sigma_g\).
  • Assign a \(\pm 1\) to each one, such that the total sum is \(e\)
  • Choose one of them to be called the main disk.
• Over \(\Sigma'\), take two parallel sections \(s_1,s_2\)
• \(s_1(\Sigma')\) and \(s_2(\Sigma')\) divide \(\pi^{-1}(\Sigma')\) in two parts: \(M_s\) and \(M_b\).
• \(M_s\cong M_b \cong \Sigma'\times I\)

Heegaard splittings of \(\mathbb{S}^1\) bundles

Over the \(D_i\), choose trivializations such that local Euler numbers are the \(\pm 1\) chosen before.

• The boundaries of the sections \(s_i\) correspond to the local curve \(-s_d\pm f_d\), so they are isotopic to the core of the solid torus.
• So we can perform float gluings of the solid tori.

Heegaard diagrams of \(\mathbb{S}^1\) bundles

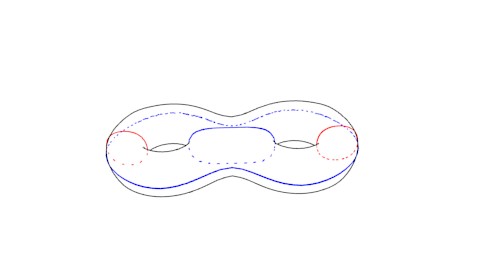
Our Heegaard surface is formed by two copies of a genus \(g\) surface, joined by \(n\) tubes.

Heegaard diagrams of \(\mathbb{S}^1\) bundles

One system of cutting curves:

Heegaard diagrams of \(\mathbb{S}^1\) bundles

The other system goes parallel except in the boundary of the floats:

Heegaard diagrams of \(\mathbb{S}^1\) bundles

The other system goes parallel except in the boundary of the floats:

Plumbing: previous lemmas

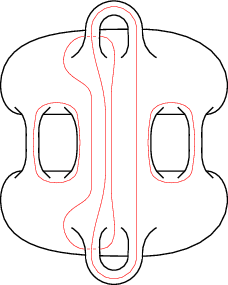
Lemma: Let \(M_1,M_2\) be handle bodies of geni \(g_1,g_2\). \(c_i\) is a curve in \(\partial M_i\) that meets only une cutting curve, transversally, and only at one point. \(A_i\) is an annulus in \(\partial M_i\) that is a regular neighborhood of \(c_i\). The gluing of \(M_1\) and \(M_2\) along an orientation-reversing homeomorphism \(A_1\to M_2\) is a handle body of genus \(g_1+g_2-1\).

Plumbing: previous lemmas

Plumbing: previous lemmas

Plumbing: previous lemmas

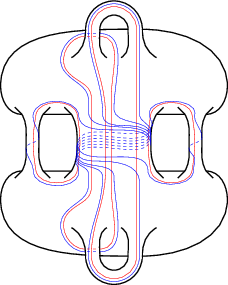
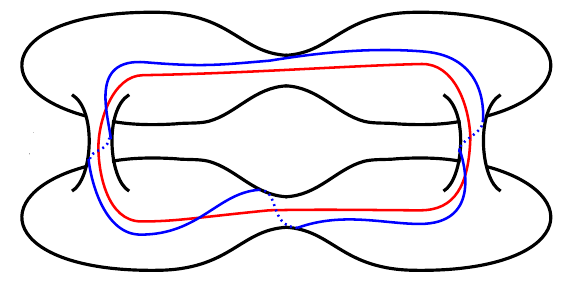
Plumbing of two handle bodies

If we have chosen the same sign in the two tubes, the pieces \(M_s,M_b\) match, so we just have to move the cutting curves in the common part until they match.

Plumbing of two handle bodies

So we have take away the cylinders of the tori being glued, and glue the cutting curves accordingly.

Plumbing of two handle bodies

One of them are just glued, the other are glued in one end, and make a turn in the other one.

Closing loops

Theorem: Let \(M\) be a handle body of genus \(g\). \(c_1,c_2\) two disjoint curves in \(\partial M\) that intersect transversally two different cutting curves in only one point each, and touch no other one. Consider \(A_1,A_2\) annuli in \(\partial M\) that are regular neighborhoods of them. The result of gluing along an orientation reversing homeomorphism \(A_1\to A_2\) is again a handle body of genus \(g\).

Closing loops

Closing loops

The resulting cutting curves are:

• One obtained by gluing the two preexisting ones
• Another one that is the commutator of a preexisting and the meridian of the gluing cylinder.

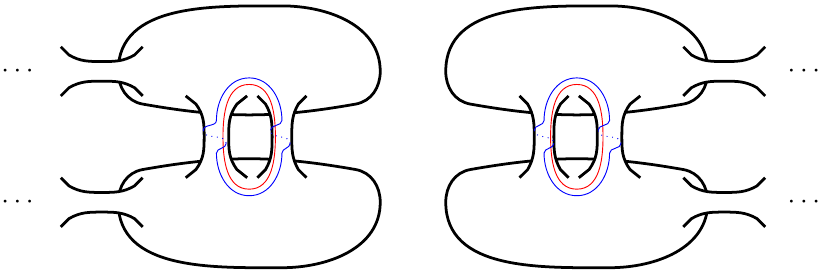
Closing loops

The resulting cutting curves are:

• One obtained by gluing the two preexisting ones
• Another one that is the commutator of a preexisting and the meridian of the gluing cylinder.

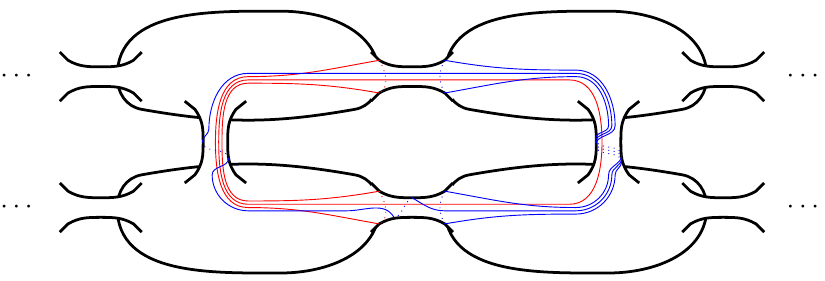
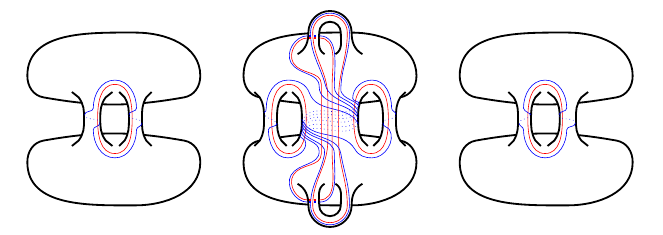
Some examples

Some examples

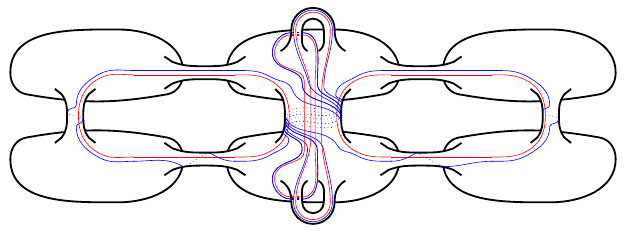
Some examples

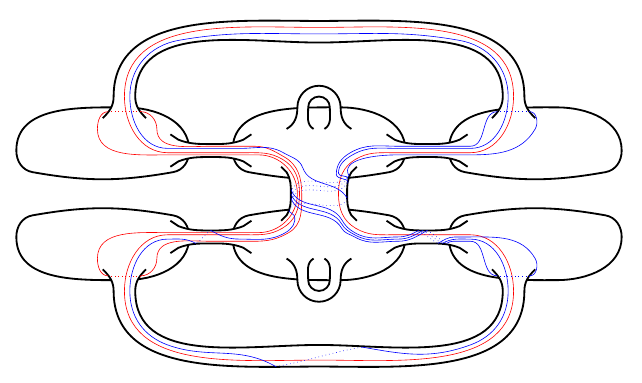
Some examples

Another examples

The Poincare sphere

Thank you!